Tags: Adaptive Learning, ALEKS, Algebra, Content, Peer-Reviewed Entry, STEM

Description
Approximately about half of students entering Science, Technology, Engineering, and Mathematics (STEM) change majors or leave college without completing a degree or certificate (Chen & Soldner, 2013). Researchers found that one of the main contributors for students to switch to non-STEM majors is the type of math courses taken in the first year (Chen & Soldner, 2013). Math is the foundation for all STEM disciplines, and the first year math experience impedes or expedites the STEM major progress and timeline (Shaw & Barbuti, 2010).
Creating quality engaging first-year math courses has been a challenge because students can differ in background, preparation or prior knowledge, and motivation. This can be even more challenging for fully online courses. Many students work and take online courses at the same time. It is imperative to accommodate students’ learning needs to support STEM retention.
Link to example artifact(s)
Course: College Algebra
Instructor: Rachid Ait Maalem Lahcen, University of Central Florida
A personalized adaptive learning strategy is adopted by the course instructor, Rachid Ait Maalem Lahcen, to improve the quality of the College Algebra course at the University of Central Florida. He integrated Keller’s Plan or the Personalized Students Instruction (PSI) (Eyre, 2007) in his course design and adopted an adaptive learning courseware, ALEKS.
PSI suggested five essential elements (Purao, Sein, Nilsen, & Larsen, 2017) that were adapted for the course design:
1. Modularized content: The instructor selected 280 topics for the course from the adaptive College Algebra curriculum. Those topics were divided into 15 learning weekly objectives or modules. Students are presented with practice problems in small chunks. The ALEKS courseware relies on adaptive questioning to accurately assess student’s knowledge state and then conveys individualized instruction. The system only presents more advanced concepts after the prerequisites are mastered.
2. Self-paced schedule: The instructor provided students with a recommended course pace for the majority to follow (one module per week) but also allowed remediation and acceleration. A student can advance to complete the course early if different milestones are achieved. This student may be able to enroll in a second math course in the same semester. A student who is engaged with the content but needs more remediation can request an adjustment of the due dates. The instructor intervenes and provides feedback if the student procrastinates or attempts topics but does not learn them.
3. Mastery is usually set to at least 80% to advance or pass a module. Frequent testing over a few modules is recommended. At the end of the course, or whenever the student learns over 80% of the 280 topics, summative or comprehensive assessment is administered. The results from this assessment is compared with the results of an initial assessment administered at the beginning of the semester to measure learning gains.
4. Supervisors or proctors: The instructor and learning/teaching assistants are essential to maintain effective human connection. Instructor-student engagement can be done using conferences, announcements, emails to provide feedback. Student-student engagement was facilitated using peer review discussions.
5. Preparation of additional course materials: The adaptive system provides student-centered learning analytics, including learning rates, login frequency and time, student progress, and learning performance. Based on the learning analytics, the instructor creates just-in-time study sets and explanations (Artifact One). If data shows that xyz topics are not mastered, the instructor provides guidance and materials to address those topics in conference meetings or in the near future activities.
Artifact One
In Spring 2019, about half of the students failed to answer the example below in a test. The system presented it as an application to solve a rational equation. However, students needed to understand the rate of completing a job prior to setting and solving the equation. The instructor prepared additional course materials to guide students as a just-in-time homework. When the rate was included as a middle step, 73% of the students answered it correctly.
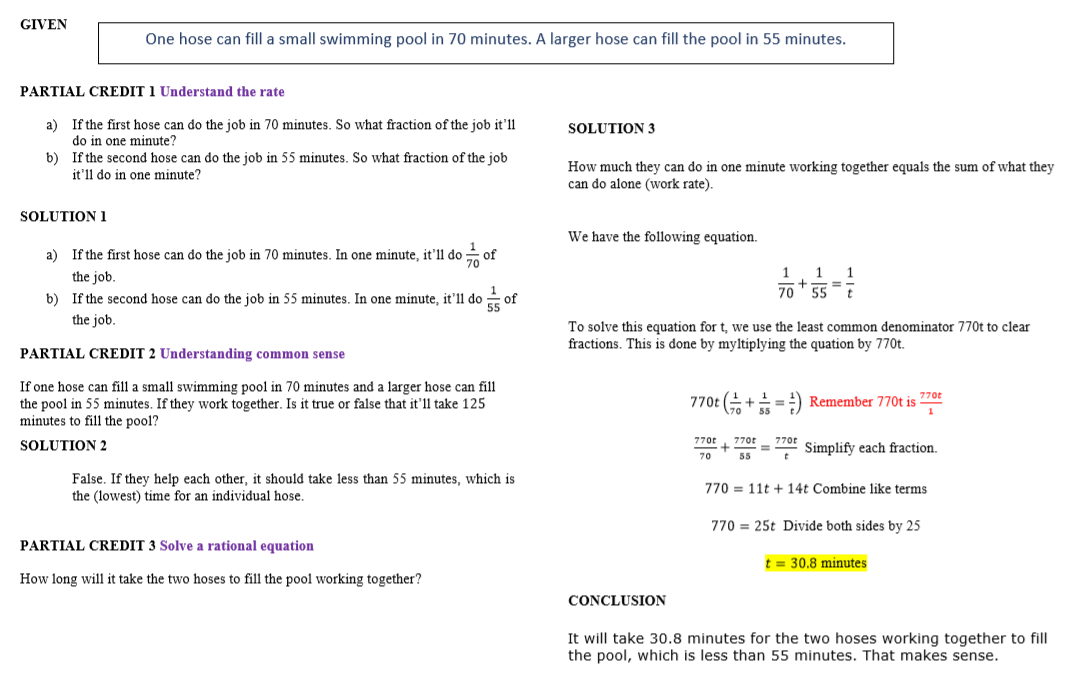
Impacts
After the first delivery of this online College Algebra course in spring 2019, the courseware analytics indicated that students’ knowledge of the targeted topics had improved by 53% with a low 6% standard deviation. The biggest gain was achieved in the hardest chapters, such as polynomial and rational functions (78%) and exponential and logarithmic functions (90%). On average, students spent 6.7 hours per week in ALEKS and their engagement gradually increased week by week towards the end of the semester. In addition, students rated the overall effectiveness of the instructor as 4.59 out of 5, which is 18% higher than the average department rating.
The adaptive strategy postulates that first-year math courses can contribute to STEM retention if students are satisfied with their learning experience, actively engaged, understand mastery requirements, and receive targeted remediation and timely feedback. This strategy is not unique to mathematics. It can be implemented to other disciplines, especially courses in the STEM fields. Other instructors could adopt the adapted PSI strategy in teaching their own adaptive courses.
Link to scholarly reference(s)
Chen, X., & Soldner, M. (2013). STEM Attrition: College Students’ Path Into and Out of STEM Fields: A Statistical Analysis Report. National Center for Education Statistics, Institute of Education Sciences, 2014–001.
Eyre, H. L. (2007). Keller’s Personalized System of Instruction: Was it a fleeting fancy or is there a revival on the horizon? The Behavior Analyst Today, 8(3), 317–324.
Purao, S., Sein, M., Nilsen, H., & Larsen, E. A. (2017). Setting the Pace: Experiments with Keller’s PSI. IEEE Transactions on Education, 60(2), 97–104.
Shaw, E. J., & Barbuti, S. (2010). Patterns of Persistence in Intended College Major with a Focus on STEM Majors. NACADA Journal, 30(2), 19–34.
Citation
Lahcen, R., & Chen, B. (2020). Adopt adaptive courseware in first year online math course to support stem retention. In A. deNoyelles, A. Albrecht, S. Bauer, & S. Wyatt (Eds.), Teaching Online Pedagogical Repository. Orlando, FL: University of Central Florida Center for Distributed Learning. https://topr.online.ucf.edu/adopting-adaptive-courseware-in-first-year-online-math-course-to-support-stem-retention/.Post Revisions:
- August 17, 2020 @ 16:58:58 [Current Revision]
- August 17, 2020 @ 16:58:58
- August 17, 2020 @ 16:48:12
- August 5, 2020 @ 14:05:03
- June 11, 2020 @ 01:26:21
- June 11, 2020 @ 01:25:42
- June 11, 2020 @ 01:25:01
- June 11, 2020 @ 01:24:02
- June 9, 2020 @ 14:38:08
- June 9, 2020 @ 14:16:57
- June 9, 2020 @ 14:15:35
- June 9, 2020 @ 14:14:51
- June 9, 2020 @ 14:14:29
- June 9, 2020 @ 14:13:44

